Illustrations of projective structures associated to maximal representations into the Lie group \(\text{Sp}(4,\mathbb{R})\).

You can click on the 3D pictures to move the veiwpoint around.

Regular octogon of hyperboloids

We first consider a simple maximal representation. We consider a Fuchsian representation of the fundamental group of a surface of genus \(2\) into \(\text{SL}(2,\mathbb{R})\) that identifies the sides of a regular hyperbolic octogon with angles \(\pi/4\). We then embed reducibly this representation via the diagonal reducible representation of \(\text{SL}(2,\mathbb{R})\) into \(\text{Sp}(4,\mathbb{R})\). The representation we obtain is maximal, and preserves a domain of discontinuity in \(\mathbb{RP}^3\).

The domain of discontinuity in this case is the complement of the black hyperboloid. This domain has two connected components. A fundamental domain for the action of our representation is obtained by removing the interior of the \(8\) colored hyperboloids. This fundamental domain can be constructed both as the boundary of a Dirichlet-Selberg domain , or by using quadrics attached to the limit map.

Hexagon of hyperboloids

We now consider any Fuchsian representation of the fundamental group of a surface of genus \(g\geq 2\) into \(\text{SL}(2,\mathbb{R})\), and we still embed reducibly this representation via the diagonal reducible representation of \(\text{SL}(2,\mathbb{R})\) into \(\text{Sp}(4,\mathbb{R})\). The representation we obtain is maximal, and preserves a domain of discontinuity in \(\mathbb{RP}^3\). We decompose the surface into hexagons by cutting along simple closed curves. The domain of discpontinuity can be decomposed into Hexagons of hyperboloids using quadrics attached to the limit map of the representation.

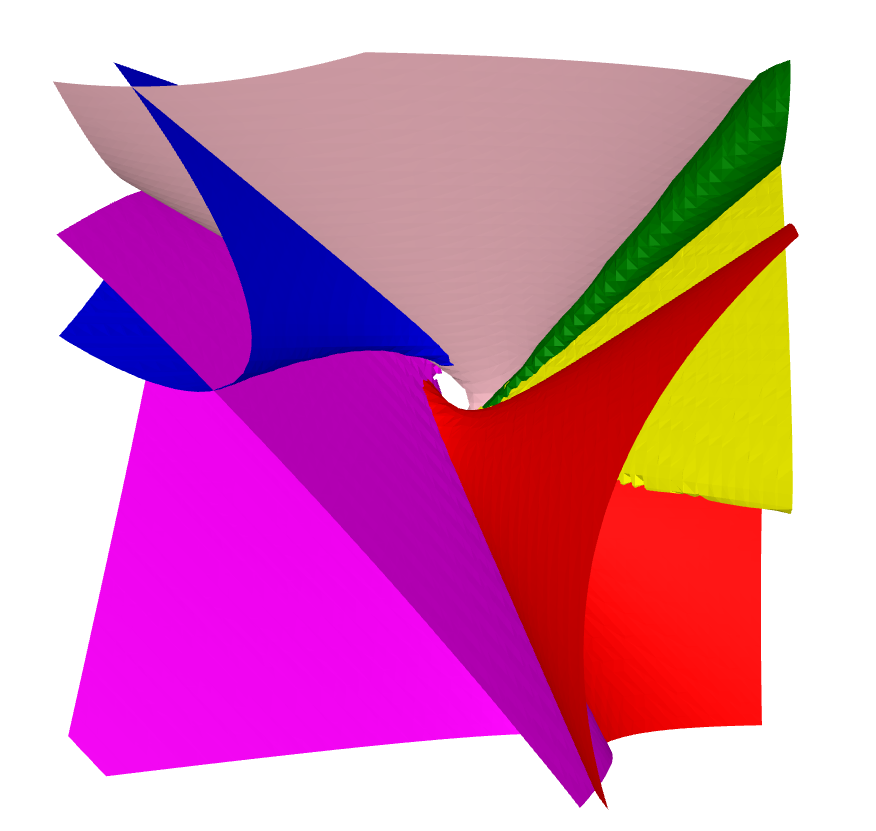
Now if we compose a Fuchsian representation by the irreducible representation of \(\text{SL}(2,\mathbb{R})\) into \(\text{Sp}(4,\mathbb{R})\), we obtain a Hitchin representation. Hitchin representations are maximal, hence this representation admits domain of discontinuity in projective space tiled by hexagons of hyperboloids. Here is an illustration of an hexagon one can obtain. Note that here the intersection of two intersecting quadrics is topologically the union of two circles, but not necessarily two projective lines, in contrast with the reducible case.

Ideal triangle of hyperboloids

Given any maximal representation of a surface group and given any maximal lamination of the surface, the domain of discontinuity can always be decomposed into ideal triangles of hyperboloids, or ideal triangles of Photons, (see the work of Mazzoli and Viaggi). All these triangles of hyperboloids are equal to the following one, up the the action of \(\text{PSp}(4,\mathbb{R})\simeq \text{SO}_o(2,3)\).

These domains bounded by hyperboloids were studued for representations of free groups by Burelle and Treib, here is their picture.